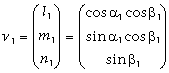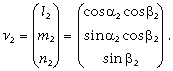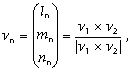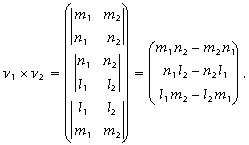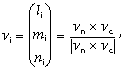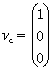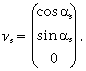Previous   |    Next

doi:10.2204/iodp.proc.314315316.122.2009

Structural geology

One of the main objectives of Expedition 315 was to document the structural geology of the uppermost ~1000 m CSF at the site proposed for deep drilling (~2.5 km) during Stage 2 of the Nankai Trough Seismogenic Zone Experiment (NanTroSEIZE). The deep drilling program will cross a major thrust in the accretionary prism (referred to as the “megasplay,” as it merges at depth with the main décollement of the subducting plate boundary), and Site C0001 was planned to provide the first information on the composition and structural architecture of the hanging wall of this major thrust. Documentation of the structures at this site is also necessary to understand the deformation history and associated geochemical and physical processes related to the growth of the prism in this area. Deformational structures, therefore, may be critical to understanding the megasplay thrust and its role in seismogenesis along this margin.

With this in mind, we tried to document each structure observed in the split cores. In many cases, X-ray CT images of whole-round (i.e., unsplit) cores were used to identify structural features and better understand their 3-D geometry. Structural data, however, were only collected on split cores. Collected data were hand logged onto a printed form at the core table and then typed into both a spreadsheet and the J-CORES database. Where possible, structural data were also corrected for rotations related to drilling.

Observations and data collection

Core observations and measurements followed previous ODP procedures (e.g., ODP Legs 131, 170, and 190) by using a modified plastic protractor (Fig. F6) and included taking notes on a descriptive core log designed and printed during the first days of the expedition (Fig. F7; see 315_STRUCTURE in “Supplementary material”). During Expedition 315, however, we also had access to CT images of the cores for the first time ever in ocean drilling, and we collected essentially all of the data from the working half, rather than the archive half, of the split core. CT images were regularly used to aid in identifying structures and defining their 3-D form and distribution in the core. Using the working half of the split core provided greater flexibility in removing—and cutting, if necessary—pieces of the core for more careful study and measurement. Thin sections, X-ray fluorescence samples, and XRD samples could also be tagged or collected as we described the core.

Orientations of planar and linear features in cored sediments were determined relative to the core axis, which represents vertical in the core reference frame, and the “double line” marked on the working half of the split core liner, which represents north (or 0° and 360°) in the core reference frame. To determine the orientation of a plane, two apparent dips of the planar feature were measured in the core reference frame and converted to a plane represented by either a strike and dip or a dip and dip direction. This conversion was accomplished using a spreadsheet, as described in “Orientation data analysis based on the spreadsheet.” This approach had the advantage of increasing data processing efficiency and reducing errors in transferring and retyping data (e.g., by using both R. Allmendinger’s Stereonet program and a spreadsheet). One apparent dip was typically represented by the intersection of the planar feature with the split face of the core and was quantified by measuring the dip direction and angle in the core reference frame. Typical apparent dip measurements had a trend of 090° or 270° and ranged in plunge from 0° to 90°. The second apparent dip was usually represented by the intersection of the planar feature and a cut or fractured surface at a high angle to the split face of the core axis. In many cases this was a surface either parallel or perpendicular to the core axis. In the former cases, the apparent dip lineation would trend 000° or 180° and plunge from 0° to 90°; in the latter cases, the trend would range from 000° to 360° and plunge 0°. Linear features observed in the cores were always associated with planar structures (typically faults or shear zones), and their orientations were determined by measuring either the trend and plunge in the core reference frame or the rake (or pitch) on the associated plane. All data were recorded on the log sheet with appropriate depths and descriptive information.

A range of planar deformation structures was observed in Expedition 315 cores. Most can be classified as primarily belonging to one of three types of structures: faults, shear zones (or deformation bands), and “vein structures.” This is not meant to imply that all structures are only one type—in fact, many structures show features characteristic of all three types—but at this preliminary stage, and with limited thin section and other microscopic analyses, we chose to use a limited number of broad categories. Of course, descriptive modifiers and sketches provide additional information as to the similarities and differences of individual structures. Faults are relatively planar, narrow zones of deformation characterized by a single band of concentrated deformation at the scale of the core and CT image. Faults also typically show clear evidence of displacement, including offset markers (e.g., bedding, burrows, or small pumice fragments), fault drag, and slickensided rough or polished surfaces. Faults are bright in CT images, suggesting a higher density than that of the adjacent matrix. However, a systematic study of deformation structures and brightness (i.e., CT number) was not undertaken, so there may well be significant variations in brightness, and therefore density, within and between various structures. Shear zones (or deformation bands) are generally wider than faults and are typically composed of multiple sets or bands of concentrated deformation. In many cases, the outer edges of a shear zone are sharp and more planar than the anastamosing surfaces of deformation within the zone. CT images also suggest a range of densities within individual zones as well as between different shear zones. Vein structures include a variety of structures, many of which have been recognized during previous ocean drilling expeditions and have been interpreted as dewatering structures. This category of structures includes the classic sigmoidal-shaped suite of thin mud-filled veins first documented by Brothers et al. (1996), Cowan (1982), and Ogawa (1980), as well as other veinlike structures that appear darker than the surrounding sediment and brighter (or denser) in CT images. These other veinlike structures include single planar to subplanar features several millimeters thick but associated with only limited displacements and rare to poorly developed slickenlines. In some cases, veinlike structures extend subparallel to the core axis for decimeters; in other cases, they occur at moderate to low angles to the core axis and have limited extent. Apparent offsets in the split core include both normal and thrust displacements. Kink bands are another type of structure observed in cored sediments, but well-developed kink bands appear to be relatively rare. In fact, only two occurrences are documented, although more detailed analysis may show that some of the structures classified as faults or deformation bands evolved from kink or kinklike structures.

Slickenlines represent the only linear structures observed in the cored sediments and are often associated with steps or, less commonly, spoon-shaped indentations on the faulted or sheared surface, producing an asymmetry that reflects the sense of slip during deformation. For a review of sense of shear criteria associated with mesoscopic brittle deformation of rocks, see Petit (1987) and Angelier (1994). Steps were interpreted as extension cracks (or “R” shears activated as extension cracks) that connect en echelon “P” shears within the fault or shear zone. In some cases, the combined set of P shears and extension cracks provides an indication of shear sense; in other cases, extension cracks or P shears alone were used as evidence for slip sense. Spoon-shaped indentations were interpreted as R shears with the fault or shear zone and indicated a sense-of-slip synthetic to the main fault or shear zone. Slickenlines and associated shear sense indictors therefore provided critical information regarding slip direction during deformation.

Paleomagnetic data were routinely used to correct drilling-induced rotations of cored sediments. Rotations were induced during RCB coring, as is common in ocean drilling, and unexpectedly with the HPCS. Rotations of the core liner used in the HPCS, including any retrieved sediments, were apparently induced as the cutting shoe was removed on the rig floor (see “Paleomagnetism”); the sense and magnitude of rotations observed in the sediments are at least consistent with this interpretation. The core liner may have also twisted and rotated as the cutting shoe was installed before coring, but this remains to be investigated. To correct for these rotations, we identified sections of cored sediment that were relatively coherent and continuous—that is, sections that were interpreted to have rotated as a single piece during drilling or core liner removal. These coherent sections were then matched with paleomagnetic data from the appropriate depth intervals and used to unrotate the planar and linear structures preserved in the core section. In cases where core sections were not continuous (generally this included core sections <10 cm in length), individual samples were placed in a core liner at 20 cm intervals, which is the sampling length of the long-core cryogenic magnetometer on the Chikyu.

Orientation data analysis based on the spreadsheet

A spreadsheet template was developed and used during Expedition 315 to calculate orientation data in both core and geographic reference frames from the linear data collected from the cores (e.g., Fig. F8; see 315_STRUCTURE in “Supplementary material”). The core reference frame is defined such that the upcore direction is vertical upward, the core-splitting plane is east–west vertical, and the double-lined side of the working half points toward north (Fig. F9).

Calculation of plane orientation

For a bedding or fault plane, two apparent dips on two different surfaces (e.g., the split core surface, which is east–west vertical, and the horizontal or north–south vertical surface) were measured in the core reference frame as azimuths (measured clockwise from north, looking down) and plunges (Fig. F10). A coordinate system was defined in such a way that the positive x-, y-, and z-directions coincide with north, east, and vertical downward, respectively (Fig. F9). If the azimuths and plunges of the two apparent dips are given as (α₁, β₁) and (α₂, β₂), respectively, as in Figure F10, then the unit vectors representing these two lines, v₁ and v₂, are

and

The unit vector normal to this plane, v_n (Fig. F10), is then defined as follows:

where

The azimuth, α_n, and plunge, β_n, of v_n are given by

α_n = tan^–1(m_n/l_n), β_n = sin^–1n_n.

The dip direction, α_d, and dip angle, β, of this plane are α_n and 90° + β_n, respectively, when β_n is <0° (Fig. F11A). They are α_n ± 180° and 90° – β_n, respectively, when β_n is ≥0° (Fig. F11B). The right-hand rule strike of this plane, α_s, is then given by α_d – 90° (Fig. F11).

Calculation of slickenline rake

For a fault with slickenlines, the apparent rake angle of the slickenline, ϕ_a, was measured on the fault surface from either the 090° or 270° direction of the split-core surface trace (Fig. F12). Fault orientation was measured as described above. Provided that v_n and v_c are unit vectors normal to the fault and split core surfaces, respectively, the unit vector of this intersection line, v, is perpendicular to both v_n and v_c (Fig. F12) and is therefore defined as follows:

where

and

Knowing the right-hand rule strike of the fault plane, α_s (Figs. F11, F13), the unit vector, v_s, toward this direction is then

The rake angle of the intersection line, ϕ_i, measured from the strike direction is given by

ϕ_i = cos^–1(v_s × v_i)

because

The rake angle of the slickenline, ϕ, from the strike direction is ϕ_i ± ϕ_a, depending on the direction the apparent rake was measured from and the direction the fault plane dips toward. ϕ_a should be subtracted from ϕ_i when the fault plane dips west and ϕ_a was measured from either the upcore or 090° direction (Fig. F13A) or when the fault plane dips toward east and ϕ_a was measured from either the downcore or 090° direction (Fig. F13B). On the other hand, ϕ_a should be added to ϕ_i when the fault plane dips toward east and ϕ_a was measured from either the upcore or 270° direction (Fig. F13C) or when the fault plane dips west and ϕ_a was measured from either the downcore or 270° direction (Fig. F13D).

Azimuth correction using paleomagnetic data

Provided that a core is vertical, its magnetization is primary, and its bedding is horizontal, its paleomagnetic declination, α_p, indicates magnetic north when its inclination, β_p, is ≥0° (Fig. F14A), whereas it indicates magnetic south when β_p is <0° (Fig. F14B). The dip direction and strike of a plane in the geographic reference frame, α_d* and α_s*, are therefore

α_d* = α_d – α_p

and

α_s* = α_s – α_p

when

β_p ≥ 0°

and are

α_d* = 180° + α_d – α_p

and

α_s* = 180° + α_s – α_p

when

β_p < 0°.

J-CORES structural database

Structural data entered in the J-CORES database VCD program include a visual (macroscopic and/or microscopic) description of core structures at a given section index and a record of planar structures in the core reference frame. Tablet PCs equipped with a wireless interface and writing recognition software were provided to allow data input directly from the core description tables. However, we found it more efficient to record the data on paper logs first (Fig. F15) and then transfer the data into the J-CORES database at a later time (see “J-CORES in practice for the structural geologist”). Data were also entered into a spreadsheet for postacquisition processing.

Data entry through the VCD program proceeds as follows:

1. Select expedition, site, hole, core, and section for which data will be read/written.
2. Select data to work with (e.g., CT image, structure description) and click Hire and OK.
3. Select a structure ID (e.g., bedding, fault) or create a new structure identifier (ID).
4. Select the section interval in which the structure is observed.
5. Fill the parameters related to this particular structure (comments and planar data; Fig. F15).

After saving, data are automatically uploaded to the Chikyu server and can be retrieved afterward as text or as an Excel file for processing. It is important to note that scientists are only allowed to write data in their own field of research and for their expedition (for example, an Expedition 315 structural geologist can only input structural data for Expedition 315). Any logged-in user has “read” access to the entire database.

J-CORES in practice for the structural geologist

Using the VCD program for real-time logging at the core table proved to be difficult for four main reasons. First, drawings are critical for structural description, yet they cannot be entered into the program via the tablet PC. Second, the handwriting recognition software is prone to errors. For example, the number 90 is commonly interpreted as the word “go,” which yields an error message in the number-only plane parameters box. This is particularly inconvenient because in a core reference frame the number 90 is used very often. Confusion also occurs between the numbers 1 and 7, which is somewhat more pernicious because it does not automatically yield an error message. Third, some numbers or combinations of numbers make the software systematically freeze, even if entered through a keyboard. Indeed, ~3% of our fault data could not be entered into J-CORES because of apparent bugs. When such bugs are encountered, the data saved in the last 10 min are usually lost because apparently their upload to the server is not instantaneous. Finally, the J-CORES VCD program is not well adapted for documenting multiple data that are linked or genetically related (e.g., fault planes, slickenlines, and sense-of-slip data; fold axes and axial planes; and bedding and current lineations). There are two options for working around this limitation while inputting fault data (Fig. F15). The first option is to use one structure ID (e.g., “normal fault”) and plane orientation and then enter the slickenline data in the comment box of the structure. In this case, the slickenline data are not exportable as an Excel spreadsheet. The second option is to enter two structure IDs (“normal fault” and “slickenline”) at the same depth interval. In this case both data may be exported, but as separate sets (i.e., the slickenline is not bound to the fault). In very deformed sections, it becomes almost impossible to know which slickenline belongs to which fault. In addition, the rake data of the slickenline must also be entered into the comment box because conversion of rake data into trend/​plunge data is not supported by the program.

During Expedition 315, J-CORES proved to be a useful tool for data comparison and integration. We used the VCD program to develop a general overview of fault and shear zone densities throughout the cores. It was also used as a quick core photo display tool, convenient for the recognition of coherent intervals appropriate for paleomagnetic reconstruction work or for a quick recall of CT scan images.

How to improve future versions of J-CORES for structural geologists

Practical experience garnered during Expedition 315 reveals a number of positive and negative aspects of J-CORES. Specific recommendations for improvement are as follows:

• Enhance the handwriting recognition software,
• Provide a means to record sketches,
• Correct the bugs related to the entry of numerical data,
• Increase the frequency and/or speed of data uploads to offset data loss as a result of crashes,
• Allow fault data to include kinematic information (i.e., lineation orientation and sense) as a single set in any format (strike, dip; dip direction, dip; strike, dip, dip quadrant; or slickenline rake or trend),
• Allow direct copy and paste of the data to and from the J-CORES database (e.g., from the J-CORES database to Excel and vice versa), and
• Reduce the number of icons to click on for one action (e.g., skip Apply before Save when entering a new structure).

Top of page   |    Previous   |    Next